When carrying out any kind of data collection or analysis, it’s essential to understand the nature of the data you’re dealing with.
Within your dataset, you’ll have different variables—and these variables can be recorded to varying degrees of precision. This is what’s known as the level of measurement.
There are four main levels of measurement: nominal, ordinal, interval, and ratio. In this guide, we’ll explain exactly what is meant by levels (also known as types or scales) of measurement within the realm of data and statistics—and why it matters. We’ll then introduce you to the four types of measurements, providing a few examples of each.
If you’d like to get your hands on some datasets already, try this free data analytics short course to get started.
Here’s what we’ll cover:
- What are levels of measurement in data and statistics?
- What are the four levels of measurement?
- Levels of measurement: FAQ
- Key takeaways
Let’s get started!
1. What are levels of measurement in data and statistics?
Level of measurement refers to how precisely a variable has been measured. When gathering data, you collect different types of information, depending on what you hope to investigate or find out.
For example, if you wanted to analyze the spending habits of people living in Tokyo, you might send out a survey to 500 people asking questions about their income, their exact location, their age, and how much they spend on various products and services. These are your variables: data that can be measured and recorded, and whose values will differ from one individual to the next.
When we talk about levels of measurement, we’re talking about how each variable is measured, and the mathematical nature of the values assigned to each variable. This, in turn, determines what type of analysis can be carried out.
Let’s imagine you want to gather data relating to people’s income. There are various levels of measurement you could use for this variable. You could ask people to provide an exact figure, or you could ask them to select their answer from a variety of ranges—for example:
- (a) 10-19k
- (b) 20-29
- (c) 30-39k
You could ask them to simply categorize their income as “high,” “medium,” or “low.”
Can you see how these levels vary in their precision? If you ask participants for an exact figure, you can calculate just how much the incomes vary across your entire dataset (for example).
However, if you only have classifications of “high,” “medium,” and “low,” you can’t see exactly how much one participant earns compared to another. You also have no concept of what salary counts as “high” and what counts as “low”—these classifications have no numerical value. As a result, the latter is a less precise level of measurement.
Why are levels of measurement important?
Level of measurement is important, as it determines the type of statistical analysis you can carry out. As a result, it affects both the nature and the depth of insights you’re able to glean from your data.
Certain statistical tests can only be performed where more precise levels of measurement have been used, so it’s essential to plan in advance how you’ll gather and measure your data.
3. What are the four levels of measurement? Nominal, ordinal, interval, and ratio scales explained
There are four types of measurement (or scales) to be aware of: nominal, ordinal, interval, and ratio.
Each scale builds on the previous, meaning that each scale not only “ticks the same boxes” as the previous scale, but also adds another level of precision.
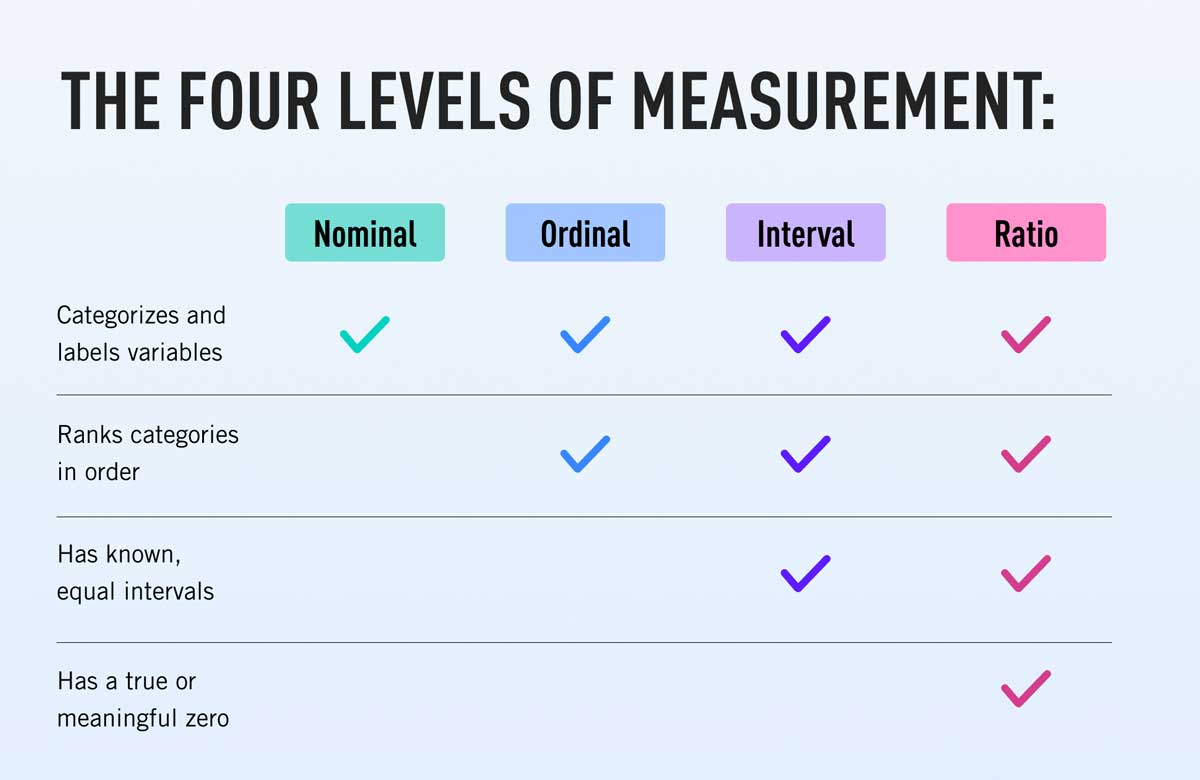
Let’s go through each in turn to give you an idea of what they are, and how they interact.
Nominal
The nominal scale simply categorizes variables according to qualitative labels (or names). These labels and groupings don’t have any order or hierarchy to them, nor do they convey any numerical value.
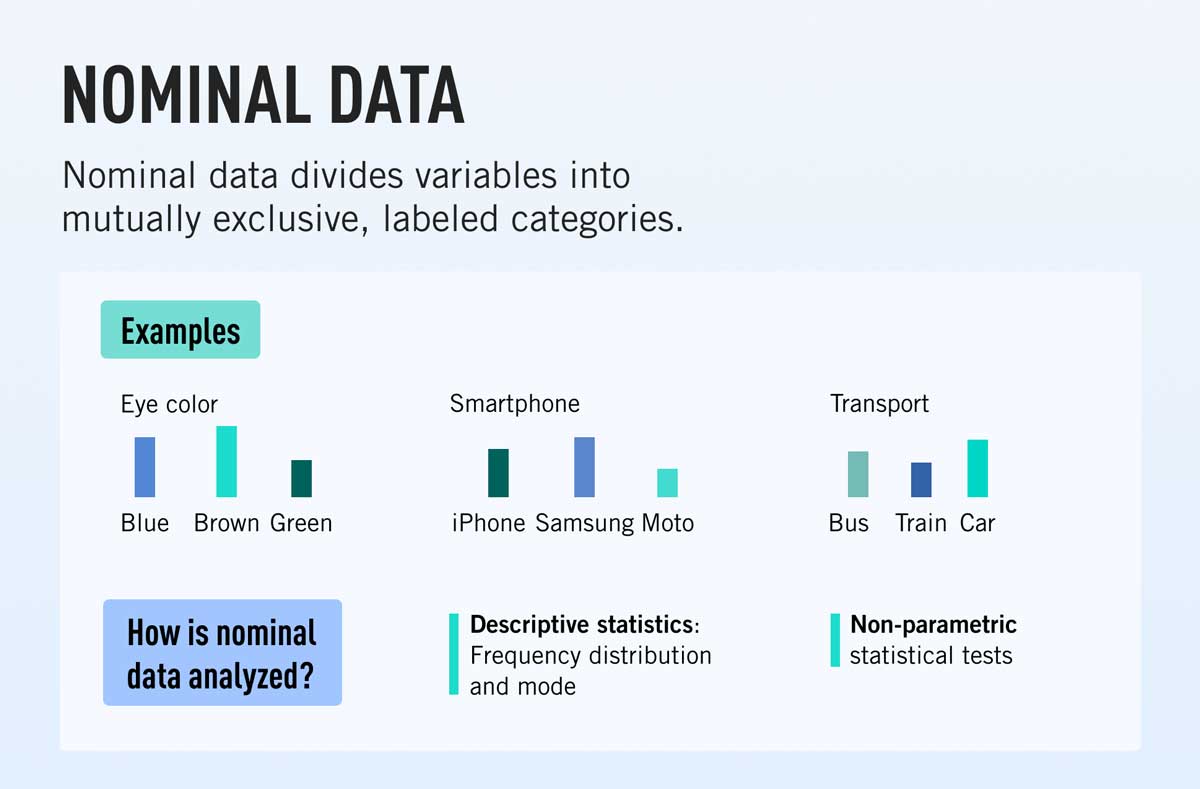
For example, the variable “hair color” could be measured on a nominal scale according to the following categories: blonde hair, brown hair, gray hair, and so on.
You can learn more in this complete guide to nominal data.
Ordinal
The ordinal scale also categorizes variables into labeled groups, and these categories have an order or hierarchy to them.
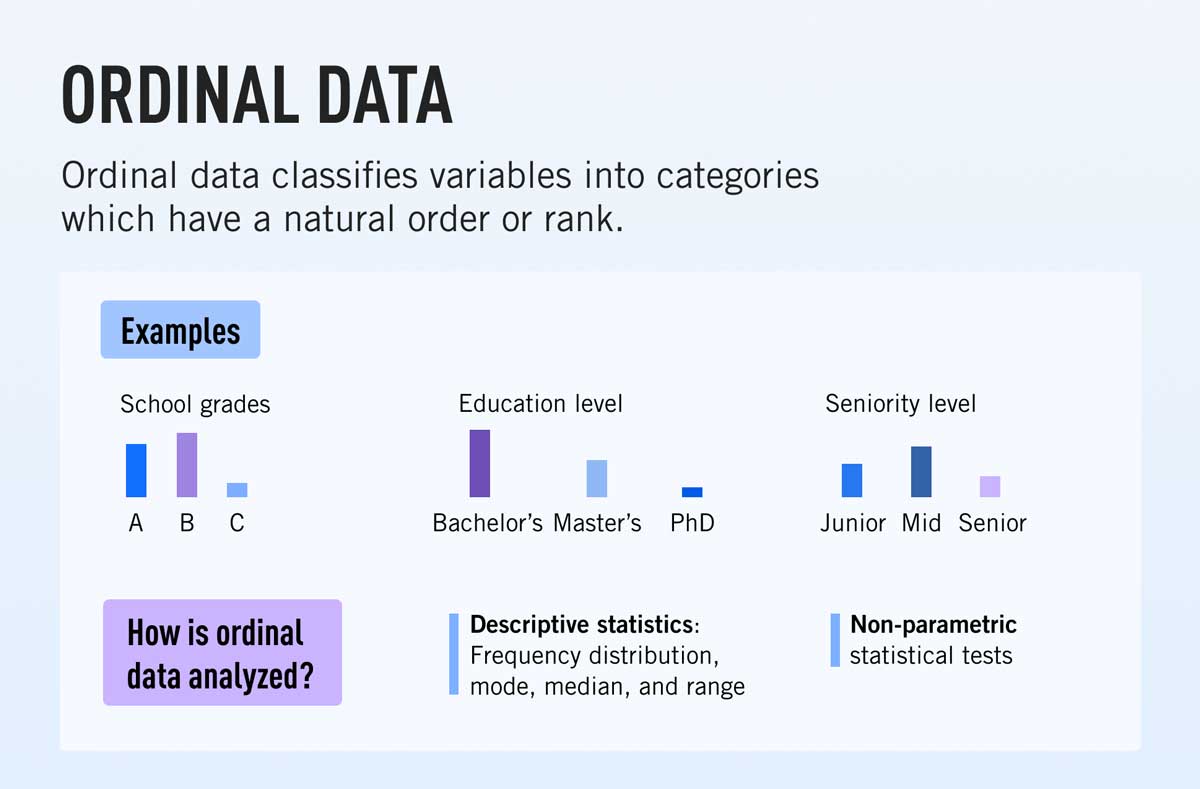
For example, you could measure the variable “income” on an ordinal scale as follows:
- low income
- medium income
- high income.
Another example could be level of education, classified as follows:
- high school
- master’s degree
- doctorate
These are still qualitative labels (as with the nominal scale), but you can see that they follow a hierarchical order.
Learn more in our guide to ordinal data.
Interval
The interval scale is a numerical scale which labels and orders variables, with a known, evenly spaced interval between each of the values.
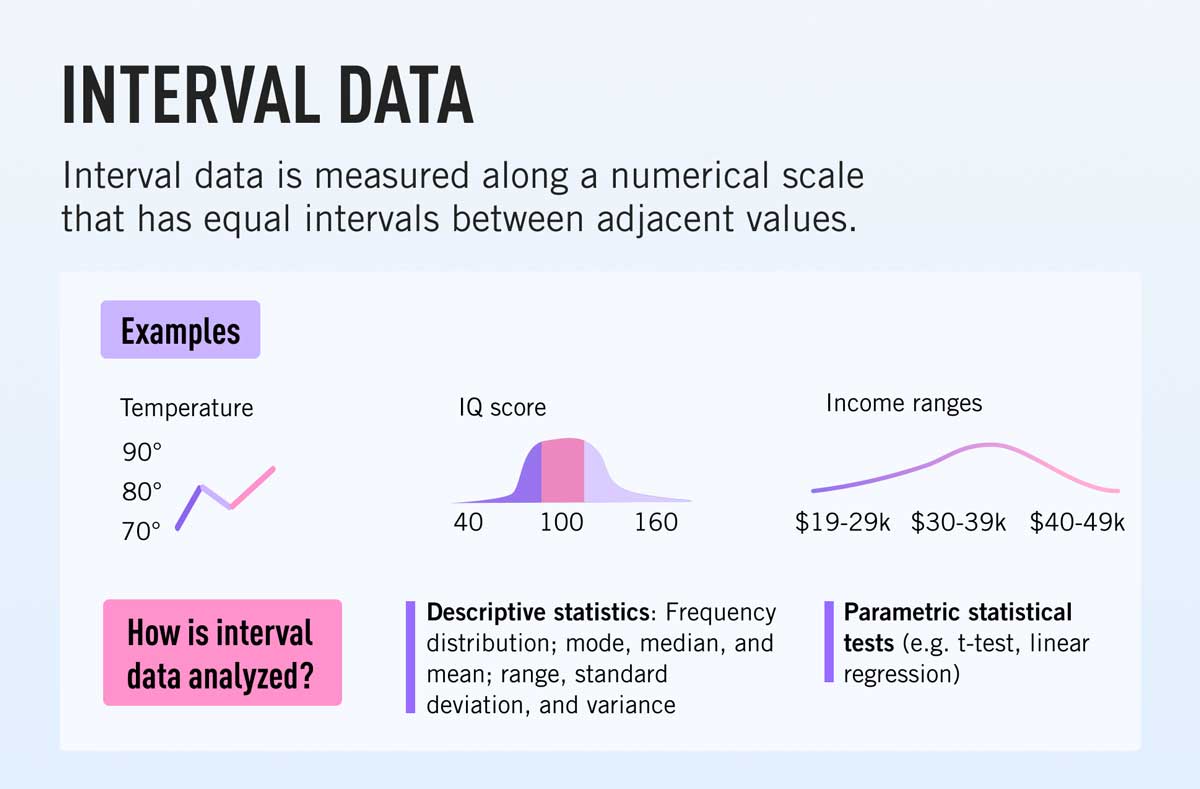
A commonly-cited example of interval data is temperature in Fahrenheit, where the difference between 10 and 20 degrees Fahrenheit is exactly the same as the difference between, say, 50 and 60 degrees Fahrenheit.
Find out more about interval data in our full guide.
Ratio
The ratio scale is exactly the same as the interval scale, with one key difference: The ratio scale has what’s known as a “true zero.”
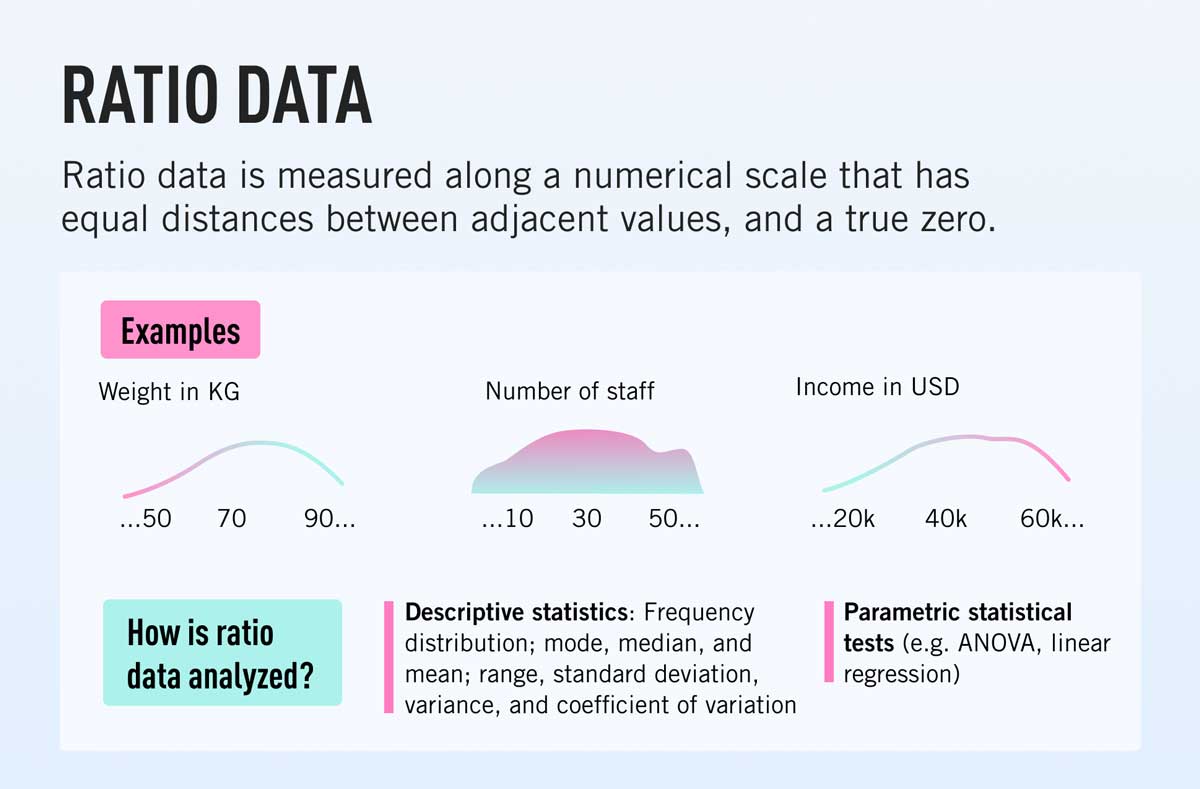
A good example of ratio data is weight in kilograms. If something weighs zero kilograms, it truly weighs nothing—compared to temperature (interval data), where a value of zero degrees doesn’t mean there is “no temperature,” it simply means it’s extremely cold!
You’ll find we’ve made a full guide to ratio data if you want to dive deeper.
4. Another way of thinking about the levels of measurement
Another way to think about levels of measurement is in terms of the relationship between the values assigned to a given variable.
With the nominal scale, there’s no relationship between the values; there’s no relationship between the categories “blonde hair” and “black hair” when looking at hair color, for example. The ratio scale, on the other hand, is very telling about the relationship between variable values.
For example, if your variable is “number of clients” (which constitutes ratio data), you know that a value of four clients is double the value of two clients. As such, you can get a much more accurate and precise understanding of the relationship between the values in mathematical terms.
In that sense, there’s an implied hierarchy to the four levels of measurement. Analysis of nominal and ordinal data tends to be less sensitive, while interval and ratio scales lend themselves to more complex statistical analysis. With that in mind, it’s generally preferable to work with interval and ratio data.
5. Levels of measurement: FAQ
What are the 4 levels of measurement?
The 4 levels of measurement, also known as measurement scales, are nominal, ordinal, interval, and ratio. These levels are used to categorize and describe data based on their characteristics and properties.
What is level of measurement in statistics?
Level of measurement, also known as scale of measurement, refers to the process of categorizing data based on the characteristics and properties of the data. It’s important in statistics because it helps determine the appropriate statistical methods and tests that can be used to analyze the data.
Is age an interval or ratio?
Age is typically considered to be measured on a ratio scale. This is because age has a true zero point, which means that a value of zero represents the absence of age. In addition, it’s possible to perform mathematical operations such as addition, subtraction, multiplication, and division on age values.
Is gender nominal or ordinal?
Gender is typically considered to be measured on a nominal scale. This is because gender is a categorical variable that has no inherent order or ranking. It’s not possible to perform mathematical operations on gender values.
5. Key takeaways
So there you have it: the four levels of data measurement and how they’re analyzed. In this article, we’ve learned the difference between the various levels of measurement, and introduced some of the different descriptive statistics and analyses that can be applied to each.
If you’re looking to pursue a career in data analytics, or even just dabbling in statistics, this fundamental knowledge of the types of measurement will stand you in good stead.
If you enjoyed learning about the different levels of measurement, why not get a hands-on introduction to data analytics with this free, 5-day short course?
At the same time, keep building on your knowledge with these guides:
